Periodicity of Solar Eclipses
Fred Espenak
1.1 Eclipse Seasons
The orbit of the Moon around Earth is inclined about 5.1° to Earth's orbit around the Sun. As a consequence, the Moon's orbit crosses the ecliptic at two points or nodes. If New Moon takes place within about 17° of a node [1], then a solar eclipse will be visible from some location on Earth.
The Sun makes one complete circuit of the ecliptic in 365.24 days, so its average angular velocity is 0.99° per day. At this rate, it takes 34.5 days for the Sun to cross the 34° wide eclipse zone centered on each node. Because the Moon's orbit with respect to the Sun has a mean duration of 29.53 days, there will always be one and possibly two solar eclipses during each 34.5-day interval when the Sun passes through the nodal eclipse zones. These time periods are called eclipse seasons.
The mid-point of each eclipse season is separated by 173.3 days which is the mean time for the Sun to travel from one node to the next. The period is a little less that half a calendar year because the lunar nodes slowly regress westward by 19.3° per year.
Footnotes
[1] The actual value ranges from 15.39° to 18.59° because of the eccentricity of the Moon's (and Earth's) orbit.
[2] The terms BCE and CE are abbreviations for "Before Common Era" and "Common Era," respectively. They are the secular equivalents to the BC and AD dating conventions. (See: Year Dating Conventions)
1.2 Interval Between Two Successive Solar Eclipses
The time interval between any two successive solar eclipses can be either 1, 5, or 6 lunations (synodic months).
Earth will experience 11,898 eclipses of the Sun during the 5000-year period from -1999 to +3000 (2000 BCE to 3000 CE [2] ). As published in in both the Five Millennium Canon of Solar Eclipses and Five Millennium Catalog of Solar Eclipses, the distribution of the 11,897 intervals between these eclipses is found in Table 1.
| Table 1 - Interval Between Successive Solar Eclipses | ||
| Number of Lunations |
Number of Eclipses |
Percent |
| 1 | 1,361 | 11.4% |
| 5 | 2,743 | 23.1% |
| 6 | 7,793 | 65.5% |
Footnotes
[1] The terms BCE and CE are abbreviations for "Before Common Era" and "Common Era," respectively. They are the secular equivalents to the BC and AD dating conventions. (See: Year Dating Conventions)
1.3 Solar Eclipse Repetition
Eclipses separated by 1, 5 or 6 lunations are usually quite dissimilar. They are often of unlike types (i.e., partial, annular, total or hybrid) with diverse Sun-Moon-Earth alignment geometries, and with different lunar orbital characteristics (i.e., longitude of perigee and longitude of ascending node). More importantly, these short periods are of no value as predictors of future eclipses because they do not repeat in any recognizable pattern.
A simple eclipse repetition cycle can be found by requiring that certain orbital parameters be repeated. The Moon must be in the new phase with the same longitude of perigee and same longitude of the ascending node. These conditions are met by searching for an integral multiple in the Moon's three major periods-the synodic, anomalistic and draconic months. A fourth condition might require that an eclipse occur at approximately the same time of year to preserve the axial tilt of Earth and thus, the same season, as well as the distance from the Sun.
1.4 Saros
The Saros arises from a harmonic between three of the Moon's orbital cycles. All three periods are subject to slow variations over long time scales, but their current values (2000 CE) are:
Synodic Month (New Moon to New Moon) = 29.530589 days = 29d 12h 44m 03s
Anomalistic Month (perigee to perigee) = 27.554550 days = 27d 13h 18m 33s
Draconic Month (node to node) = 27.212221 days = 27d 05h 05m 36s
One Saros is equal to 223 synodic months, however, 239 anomalistic months and 242 draconic months are also equal (within a few hours) to this same period:
223 Synodic Months = 6585.3223 days = 6585d 07h 43m
239 Anomalistic Months = 6585.5375 days = 6585d 12h 54m
242 Draconic Months = 6585.3575 days = 6585d 08h 35m
With a period of approximately 6,585.32 days (~18 years 11 days 8 hours), the Saros is valuable tool in investigating the periodicity and recurrence of eclipses. It was first known to the Chaldeans as an interval when lunar eclipses repeat, but the Saros is applicable to solar eclipses as well.
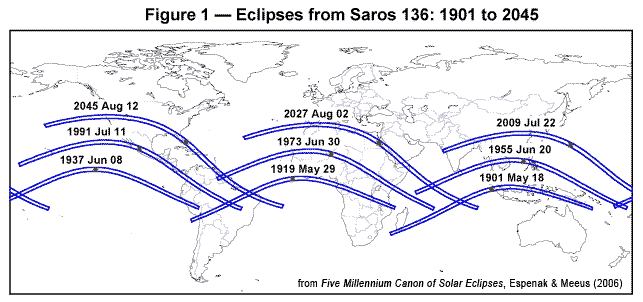
Figure 1 - Eclipses from Saros 136: 1901 to 2045
(click for larger figure)
Any two eclipses separated by one Saros cycle share similar characteristics. They occur at the same node with the Moon at nearly the same distance from Earth and at the same time of year. Because the Saros period is not equal to a whole number of days, its biggest drawback as an eclipse predictor is that subsequent eclipses are visible from different parts of the globe. The extra 1/3 day displacement means that Earth must rotate an additional ~8 hours or ~120° with each cycle. For solar eclipses, this results in a shift of each succeeding eclipse path by ~120° west. Thus, a Saros series returns to approximately the same geographic region every three Saros periods (~54 years and 34 days). This triple Saros cycle is known as the Exeligmos. Figure 1 shows the path of totality for nine eclipses belonging to Saros 136. This series is of particular interest because it is currently producing the longest total eclipses of the 20th and 21st centuries. The westward migration of each eclipse path from 1901 through 2045 illustrates the consequences of the extra 1/3 day in the Saros period. The northward shift of each path is due to the progressive increase in gamma from -0.3626 (in 1901) to 0.2116 (in 2045). The color figure Recent Eclipses of Saros 136 shows nine eclipse paths from the series (1937 through 2081).
Saros series do not last indefinitely because the synodic, draconic, and anomalistic months are not perfectly commensurate with one another. In particular, the Moon's node shifts eastward by about 0.48° with each eclipse in a series. The following narrative describes the life cycle of a typical Saros series at the Moon's descending node. The series begins when the New Moon occurs ~17° east of the node. The Moon's umbral/antumbral shadow passes about 3500 km south of Earth and a small partial eclipse will be visible from high southern latitudes. One Saros period later, the umbra/antumbra passes ~250 km closer to Earth's geocenter (gamma increases) and a partial eclipse of slightly larger magnitude will result. After about 10 Saros cycles (~200 years), the first umbral/antumbral eclipse occurs near the South Pole of Earth. Over the course of the next 7 to 10 centuries, a central eclipse occurs every 18.031 years (= Saros), but will be displaced northward by about 250 km with respect to Earth's center. Halfway through this period, eclipses of long duration occur near the equator (mid-series eclipses may be of short duration if hybrid or nearly so). The last central eclipse of the series takes place at high northern latitudes. Approximately 10 more eclipses will be partial with successively smaller magnitudes. Finally, the Saros series ends 12 to 15 centuries after it began at the opposite pole.
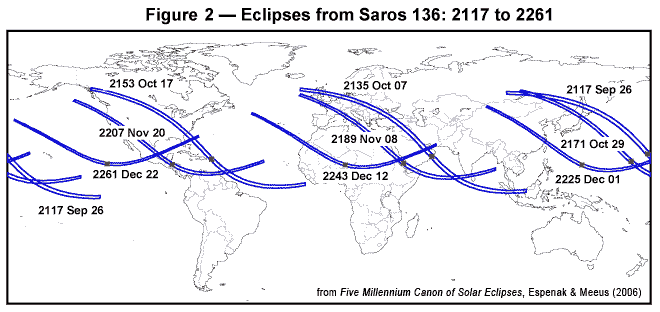
Figure 2 - Eclipses from Saros 136: 2117 to 2261
(click for larger figure)
Based on the above description, the path of each umbral/antumbral eclipse should shift uniformly north in latitude after every Saros period. As Fig. 2 shows, this is not always the case. Nine members from Saros 136 are plotted for the years 2117 through 2261. Although the paths of previous eclipses in this series were shifting progressively northward (Figure 1), the trend here is reversed and the paths shift south. This temporary effect is due to the tilt of Earth's axis combined with the passage of Saros 136 eclipses from the Northern Hemisphere's autumnal equinox through winter solstice. Note that the season for this group of eclipses runs from September through December. With each successive eclipse, Earth's Northern Hemisphere tips further and further away from the Sun. This motion shifts geographic features and circles of latitude northward with respect to the Sun-Earth line at a rate that is faster than the change in gamma. Consequently, the eclipse paths appear to shift south in latitude until the winter solstice when they again resume a northward trend.
The scenario for a Saros series at the ascending node is similar except that gamma decreases as each successive eclipse shifts south of the previous one. The southern latitude trend in eclipse paths reverses to the north near the Northern Hemisphere summer solstice.
Because of the ellipticity of the orbits of Earth and the Moon, the exact duration and number of eclipses in a complete Saros series is not constant. A series may last 1,226 to 1,551 years and is composed of 69 to 87 eclipses, of which 39 to 59 are umbral/antumbral (i.e., annular, total, or hybrid). At present (2008), there are 39 active Saros series numbered 117 to 155. The number of eclipses in each of these series ranges from 70 to 82, however, the majority of the series (84.6%) are composed of 70 to 73 eclipses.
Historically speaking, the word Saros derives from the Babylonian term "sar" which is an interval of 3600 years. It was never used as an eclipse period until English astronomer Edmund Halley adopted it in 1691. According to R. H. van Gent, Halley "...extracted it from the lexicon of the 11th-century Byzantine scholar Suidas who in turn erroneously linked it to an (unnamed) 223-month Babylonian eclipse period mentioned by Pliny the Elder (Naturalis Historia II.10[56])."
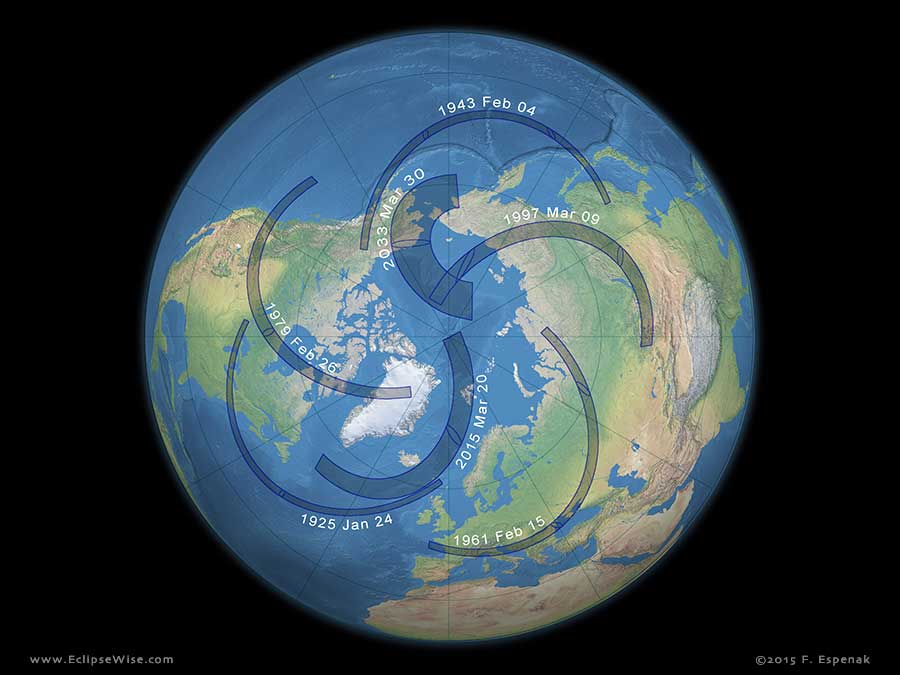
Figure 3 - The Last Seven Total Eclipses from Saros 120: 1925 to 2033
(click for larger figure)
1.5 Gamma and Saros Series
Gamma changes monotonically throughout any single Saros series. The change in gamma is larger when Earth is near its aphelion (June to July) than when it is near perihelion (December to January). For odd numbered series (ascending node), gamma decreases, while for even numbered series (descending node), gamma increases. This simple rule describes the current behavior of gamma, but this has not always been the case. The eccentricity of Earth's orbit is presently 0.0167, and is slowly decreasing. It was 0.0181 in the year -2000 and will be 0.0163 in +3000. In the past when the eccentricity was larger, there were Saros series in which the trend in gamma reversed for a few cycles before resuming its original direction. These instances occur near perihelion when the Sun's apparent motion is highest and may, in fact, overtake the eastward shift of the node. The resulting effect is a relative shift west of the node after one Saros cycle instead of the usual eastward shift. Consequently, gamma reverses direction.
The most unusual case of this occurs in Saros series 0. It began in -2955 with 11 partial eclipses, followed by 1 total, 1 hybrid, and 4 annulars. Gamma increased with each eclipse until it reversed direction with the second annular. It continued to decrease and the series began to once again produce partial eclipses. With the third partial eclipse, gamma resumed its original northward shift. The series went on to produce 45 more annular eclipses before ending in the year -1675 after 7 partial eclipses.
Among several hundred Saros series examined (-34 to 247), there are many other examples of temporary shifts in the monotonic nature of gamma, although none as bizarre as Saros 0. Some series have two separate reversals in gamma (e.g., series 15, 34, and 52) or even three (e.g., series -5 and 13). The most recent eclipse with a gamma reversal was in 1674 (Saros 107). The next and last in the Five Millennium Canon and Five Millennium Catalog will occur in 2290 (Saros 165). In past millennia, the gamma reversals were more frequent because Earth's orbital eccentricity was larger.
1.6 Saros Series Statistics
Eclipses belonging to 204 different Saros series fall within the five millennium span of the Canon and Catalog . Two series (-13 and 190) have only one or two members represented, while 81 have a larger but incomplete subset of their members included (-12 to -26, 30, 145, 147, and 151 to 189). Finally, 121 complete Saros series are contained within the Canon and Catalog (27 to 29, 31 to 144, 146, and 148 to 150).
The number of eclipses in each of these series ranges from 69 to 87; however, over a quarter (27.9%) of the series contain 72 eclipses while nearly three quarters (72.1%) of them have 70 to 73 eclipses. Table 2 presents the statistical distribution of the number of eclipses in each Saros series. The approximate duration (years) as a function of the number of eclipses is listed along with the first five Saros series containing the corresponding number of eclipses.
| Table 2 - Number of Solar Eclipses in Saros Series | |||
| Number of Solar Eclipses |
Duration (years) |
Saros Series Numbers | Saros Series |
| 69 | 1226 | 4 | 156, 171, 174, 177 |
| 70 | 1244 | 25 | 104, 116, 122, 123, 131,... |
| 71 | 1262 | 40 | 22, 25, 61, 62, 64,... |
| 72 | 1280 | 57 | -11, 0, 1, 3, 4,... |
| 73 | 1298 | 25 | -13, -12, -3, 2, 5,... |
| 74 | 1316 | 10 | -8, -1, 9, 17, 31,... |
| 75 | 1334 | 8 | -10, -9, -2, 15, 74,... |
| 76 | 1352 | 3 | 11, 108, 146 |
| 77 | 1370 | 3 | 145, 166, 184 |
| 78 | 1388 | 1 | 69 |
| 79 | 1406 | 2 | 111, 182 |
| 80 | 1424 | 4 | -4, 129, 147, 164 |
| 81 | 1442 | 1 | 109 |
| 82 | 1460 | 2 | 71, 127 |
| 83 | 1478 | 4 | 30, 72, 88, 90 |
| 84 | 1496 | 5 | 32, 33, 35, 53, 70 |
| 85 | 1514 | 4 | 13, 14, 16, 51 |
| 86 | 1533 | 5 | -7, -5, 12, 34, 52 |
| 87 | 1551 | 1 | -6 |
All Saros series begin and end with a number of partial eclipses. Among the 204 Saros series with members falling within the scope of the Canon and Catalog, the number of partial eclipses in the initial phase ranges from 6 to 25. Similarly, the number of partial eclipses in the final phase varies from 6 to 24. The middle life of a Saros series is composed of umbral/antumbral eclipses (i.e., annular, total, or hybrid), which range in number from 39 to 59. Table 3 presents the statistical distribution in the number of umbral/antumbral eclipses in the Saros series represented in the Canon and Catalog .
Saros 0 is an exception to the above scheme. After beginning with 11 partial eclipses, Saros 0 proceeds with a total, a hybrid, and an annular eclipse. The series then reverts back to three more partial eclipses. It finally resumes with a string of 45 annular eclipses before ending with 7 partial eclipses. This odd behavior is due to the higher orbital eccentricity of Earth in the past and fortuitous timing.
| Table 3 - Number of A/T/H Solar Eclipses in Saros Series | |||
| Number of A/T/H Eclipses |
Duration (years) |
Number of Series | Saros Series Numbers |
| 39 | 703 | 4 | 110, 144, 162, 165 |
| 40 | 721 | 19 | -6, 31, 34, 37,... |
| 41 | 739 | 21 | -9, -3, 12, 13,... |
| 42 | 757 | 17 | 10, 15, 16, 28,... |
| 43 | 775 | 30 | -8, -7, -5, -4,... |
| 44 | 793 | 18 | -2, 11, 17, 18,... |
| 45 | 811 | 7 | -12, 29, 48, 77,... |
| 46 | 829 | 3 | -10, 114, 151 |
| 47 | 847 | 1 | 140 |
| 48 | 865 | 6 | -1, 38, 66, 171, 188 |
| 49 | 883 | 2 | 27, 153 |
| 50 | 902 | 1 | 103 |
| 51 | 920 | 1 | 0 [3], 190 |
| 52 | 938 | 4 | 57, 64, 156, 189 |
| 53 | 956 | 8 | 40, 101, 116, 133,... |
| 54 | 974 | 6 | 47, 98, 119, 134,... |
| 55 | 992 | 14 | 43, 59, 82, 83,... |
| 56 | 1010 | 17 | -11, 1, 6, 8,... |
| 57 | 1028 | 13 | 3, 4, 7, 20,... |
| 58 | 1046 | 10 | -13, 2, 21, 26,... |
| 59 | 1064 | 2 | 5, 23 |
[3] The duration of the A/T/H eclipse sequence of Saros 0 is 974 years because it contains three partial eclipses.
A concise summary of all 204 Saros series (-13 to 190) is presented in:
Table 4: Summary of Solar Eclipses in Saros Series -13 to 190
The number of eclipses in each series is listed followed by the calendar dates of the first and last eclipses in the Saros. Finally, the chronological sequence of eclipse types in the series is tabulated. The number and type of eclipses varies from one Saros series to the next as reflected in the sequence diversity. Note that the tables make no distinction between central and non-central umbral/antumbral eclipses. The following abbreviations are used in the eclipse sequences:
P = Partial Eclipse
A = Annular Eclipse
T = Total Eclipse
H = Hybrid Eclipse
The Catalog of Solar Eclipse Saros Series contains links to 181 web pages, each one listing the details of all eclipses in a particular Saros series:
Catalog of Solar Eclipse Saros Series
1.7 Saros and Other Periods
The numbering system used for the Saros series was introduced by van den Bergh in his book Periodicity and Variation of Solar (and Lunar) Eclipses (1955). He assigned the number 1 to a pair of solar and lunar eclipse series that were in progress during the second millennium BCE based on an extrapolation from von Oppolzer's Canon der Finsternisse (1887).
There is an interval of 1, 5, or 6 synodic months between any sequential pair of solar eclipses. Interestingly, the number of lunations between two eclipses permits the determination of the Saros series number of the second eclipse when the Saros series number of the first eclipse is known. Let the Saros series number of the first eclipse in a pair be "s". The Saros series number of the second eclipse can be found from the relationships in Table 5 (Meeus, Grosjean, and Vanderleen, 1966).
| Table 5 - Some Eclipse Periods and Their Relationships to the Saros Number | |||
| Number of Synodic Months |
Length of Time | Saros Series Number | Period Name |
| 1 | ~1 month | s + 38 | Lunation |
| 5 | ~5 months | s - 33 | Short Semester |
| 6 | ~6 months | s + 5 | Semester |
| 135 | ~11 years - 1 month | s + 1 | Tritos |
| 223 | ~18 years + 11 days | s | Saros |
| 235 | ~19 years | s + 10 | Metonic Cycle |
| 358 | ~29 years - 20 days | s + 1 | Inex |
| 669 | ~54 years + 33 days | s | Exeligmos (Triple Saros) |
For more information on these periods and others, see R. H. van Gent's extensive Catalogue of Eclipse Cycles, and Felix Verbelen's Saros, Inex and Eclipse Cycles.
1.8 Saros and Inex
A number of different eclipse cycles were investigated by van den Bergh, but the most useful were the Saros and the Inex. The Inex is equal to 358 synodic months (~29 years less 20 days), which is very nearly 388.5 draconic months.
358 Synodic Months = 10,571.9509 days = 10,571d 22h 49m
388.5 Draconic Months = 10,571.9479 days = 10,571d 22h 55m
The extra 0.5 in the number of draconic months means that eclipses separated by one Inex period occur at opposite nodes. Consequently, an eclipse visible from the Northern Hemisphere will be followed one Inex later by an eclipse visible from the Southern Hemisphere, and vice versa. The Inex is equal to ~383.67 anomalistic months, which is far from an integer number. Thus, eclipses separated by one Inex will very likely be of different types, especially if they are central (i.e., total or annular).
The mean time difference between 358 synodic months and 388.5 draconic months making up an Inex is only 6 min. In comparison, the mean difference between these two cycles in the Saros is 52 min. This means that after one Inex, the shift of the Moon with respect to the node (+0.04°) is much smaller than for the Saros (-0.48°). While a Saros series lasts 12 to 15 centuries, an Inex series typically lasts 225 centuries and contains about 780 eclipses.
1.9 Saros-Inex Panorama
Van den Bergh placed all 8,000 solar eclipses in von Oppolzer's Canon der Finsternisse (1887) into a large two-dimensional matrix. Each Saros series was arranged as a separate column containing every eclipse in chronological order. The individual Saros columns were then staggered so that the horizontal rows each corresponded to different Inex series. This "Saros-Inex Panorama" proved useful in organizing eclipses. For instance, one step down in the panorama is a change of one Saros period (6585.32 days) later, while one step to the right is a change of one Inex period (10571.95 days) later. The rows and columns were then numbered with the Saros and Inex numbers.
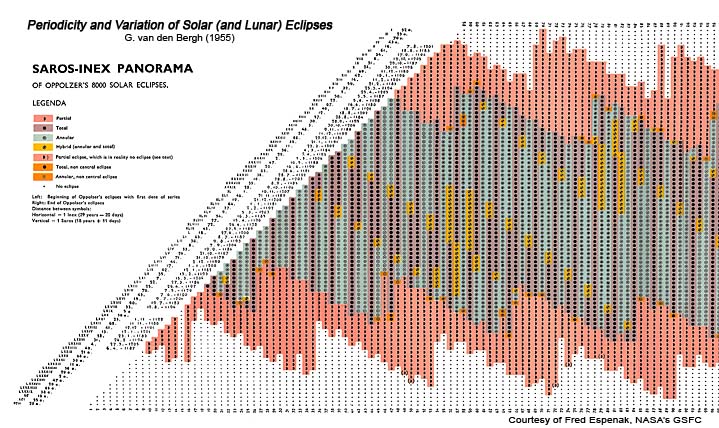
Saros-Inex Panorama of Solar Eclipses
(click for larger figure)
The panorama also made it possible to predict the approximate circumstances of solar (and lunar) eclipses occurring before or after the period spanned by von Oppolzer's Canon. The time interval "t" between any two solar eclipses can be found through an integer combination of Saros and Inex periods via the following relationship:
t = a * i + b * s (1)
where
t = interval in days,
i = Inex period of 10571.95 days (358 synodic months),
s = Saros period of 6585.32 days (223 synodic months), and
a, b = integers (negative, zero, or positive).
From this equation, a number of useful combinations of Inex and Saros periods can be employed to extend von Oppolzer's Canon from -1207 back to -1600 using nothing more than simple arithmetic (van den Bergh, 1954). The ultimate goal of the effort was to a produce an eclipse canon for dating historical events prior to -1207. Periods formed by various combinations of Inex and Saros were evaluated in order to satisfy one or more of the following conditions:
- The deviation from a multiple of 0.5 draconic months should be small
(i.e., Moon should be nearly the same distance from the node). - The deviation from an integral multiple of anomalistic months should be small
(i.e., Moon should be nearly the same distance from Earth). - The deviation from an integral multiple of anomalistic years should be small
(i.e., eclipse should occur on nearly the same calendar date).
No single Inex-Saros combination meets all three criteria, but there are periods that do a reasonably good job for any one of them. Note that secular changes in the Moon's elements cause a particular period to be of high accuracy for a limited number of centuries. The direct application of the Saros-Inex panorama allows for the determination of eclipse dates in the past (or future); however, the application of the longer Saros-Inex combinations permit the rapid estimation of a number of eclipse characteristics without lengthy calculations. Table 6 lists several of the most useful periods.
| Table 6 - Some Useful Eclipse Periods | |||
| Period Name | Period (Inex + Saros) |
Period (years) |
Use |
| Heliotrope | 58i + 6s | 1,787 | Geographic longitude of central line |
| Accuratissima | 58i + 9s | 1,841 | Geographic latitude of central line |
| Horologia | 110i + 7s | 3,310 | Time of ecliptic conjunction |
Modern digital computers using high precision solar and lunar ephemerides can directly predict the dates and circumstances of eclipses. Nevertheless, the Saros and Inex cycles remain useful tools in understanding the periodicity and frequency of eclipses.
An enormous Saros-Inex panorama has been produced by Luca Quaglia and John Tilley in the form of a Microsoft Excel file. It shows 61,775 solar eclipses over a 26,000-year period from -11,000 to +15,000 organized by Saros and Inex Series.
1.10 Secular Variations in the Saros and Inex
Because of long secular variations in the average ellipticity of the Moon's and Earth's orbits, the mean lengths of the synodic, draconic, and anomalistic months are slowly changing. The mean synodic and draconic months are increasing by approximately 0.2 and 0.4 s per millennium, respectively. Meanwhile, the anomalistic month is decreasing by about 0.8 s per millennium.
Although small, the cumulative effects of such changes has an impact on both the Saros and Inex. Table 7 shows how the number of draconic and anomalistic months change with respect to 223 synodic months (Saros period) over an interval of 7000 years. Of particular interest is the last column, which shows the mean shift of the Moon's node after a period of 1 Saros. It is gradually increasing, which means that the average number of eclipses in a typical Saros series is decreasing.
| Table 7 - Number of Anomalistic and Draconic Months in 1 Saros | |||
| Year | Anomalistic Months (223 Lunations) |
Draconic Months (223 Lunations) |
Node Shift (after 1 Saros) |
| -3000 | 238.991679 | 241.998742 | 0.4529 |
| -2000 | 238.991763 | 241.998730 | 0.4571 |
| -1000 | 238.991854 | 241.998717 | 0.4618 |
| 0 | 238.991950 | 241.998703 | 0.4668 |
| 1000 | 238.992051 | 241.998688 | 0.4722 |
| 2000 | 238.992157 | 241.998673 | 0.4779 |
| 3000 | 238.992267 | 241.998656 | 0.4838 |
| 4000 | 238.992379 | 241.998639 | 0.4899 |
Table 8 shows how the number of draconic months is changing with respect to 358 synodic months (Inex period) over a 7000-year interval. The mean shift in the lunar node after 1 Inex is much smaller than the Saros and is gradually decreasing. This explains why the lifetime of the Inex is so much longer than the Saros and is still increasing.
| Table 8 - Number of Draconic Months in 1 Inex | ||
| Year | Draconic Months (358 Lunations) |
Node Shift (after 1 Inex) |
| -3000 | 388.500223 | -0.0801 |
| -2000 | 388.500204 | -0.0734 |
| -1000 | 388.500183 | -0.0659 |
| 0 | 388.500160 | -0.0578 |
| 1000 | 388.500136 | -0.0491 |
| 2000 | 388.500111 | -0.0400 |
| 3000 | 388.500085 | -0.0305 |
| 4000 | 388.500057 | -0.0207 |
Although the Inex possesses a long lifespan, its mean duration is not easily characterized because of the decreasing nodal shift seen in Table 8. If the instantaneous mean durations of the synodic and draconic months for the years -2000, +2000, and +4000 are used to calculate the mean duration of the Inex, the resulting lengths are about 14,500, 26,600, and 51,000 years, respectively (Meeus, 2004).
Acknowledgments
The information presented on this web page is based on material originally published in Five Millennium Canon of Solar Eclipses: -1999 to +3000 and Five Millennium Catalog of Solar Eclipses: -1999 to +3000.
Permission is freely granted to reproduce this data when accompanied by an acknowledgment:
"Eclipse Predictions by Fred Espenak (NASA's GSFC) and Jean Meeus"
References
Espenak, F., and Meeus, J., Five Millennium Canon of Solar Eclipses: -1999 to +3000 (2000 BCE to 3000 CE), NASA Tech. Pub. 2006-214141, NASA Goddard Space Flight Center, Greenbelt, Maryland (2006).
Espenak, F., and Meeus, J., Five Millennium Catalog of Solar Eclipses: -1999 to +3000 (2000 BCE to 3000 CE), NASA Tech. Pub. 2008-214170, NASA Goddard Space Flight Center, Greenbelt, Maryland (2008).
Gingerich, O., (Translator), Canon of Eclipses, Dover Publications, New York (1962) (from the original T.R. von Oppolzer, book, Canon der Finsternisse, Wien, [1887]).
Meeus, J., Mathematical Astronomy Morsels III, Willmann-Bell, pp. 109-111, (2004).
Meeus, J., Grosjean, C.C., and Vanderleen, W., Canon of Solar Eclipses, Pergamon Press, Oxford, United Kingdom (1966).
van den Bergh, G., Periodicity and Variation of Solar (and Lunar) Eclipses, Tjeenk Willink, and Haarlem, Netherlands (1955).
von Oppolzer, T.R., Canon der Finsternisse, Wien, (1887).
Return to:
Six Millennium Catalog of Solar Eclipses
Catalog of Solar Eclipse Saros Series
Eclipses and the Moon's Orbit
Periodicity of Lunar Eclipses
Eclipses and the Saros
Links to Additional Solar Eclipse Predictions
- Home - home page of EclipseWise with predictions for both solar and lunar eclipses
- Solar Eclipses - primary page for solar eclipse predictions
- Solar Eclipse Links - detailed directory of links
- Six Millennium Catalog of Solar Eclipses - covers the years -2999 to +3000 (3000 BCE to 3000 CE)
- World Atlas of Solar Eclipse Maps - index page for world eclipse maps covering 5 millennia
- Javascript Solar Eclipse Explorer - calculate all solar eclipses visible from a city
- Thousand Year Canon of Solar Eclipses 1501 to 2500 - link to the publication
- MrEclipse.com - eclipse resources and tips on photography
- Solar Eclipses for Beginners - a primer on solar eclipse basics
- How to Photograph a Solar Eclipse - instructions for imaging an eclipse of the Sun
- MrEclipse Photo Index - an index of solar eclipse photographs
Acknowledgments
Some of the content on this web site is based on the book Thousand Year Canon of Solar Eclipses 1501 to 2500. All eclipse calculations are by Fred Espenak, and he assumes full responsibility for their accuracy. Permission is granted to reproduce eclipse data when accompanied by a link to this page and an acknowledgment:
"Eclipse Predictions by Fred Espenak, www.EclipseWise.com"
The use of diagrams and maps is permitted provided that they are NOT altered (except for re-sizing) and the embedded credit line is NOT removed or covered.




