Google Eclipse Map Page

Total Solar Eclipse of 2882 Mar 10

Fred Espenak

Introduction

This page features an interactive Google map [1] showing the regional visibility of the Total Solar Eclipse of 2882 Mar 10 . The Google map allows the user to zoom and scroll the map as desired. Clicking the cursor on any location will immediately generate a prediction of the eclipse circumstances from that location. The small global map to the right is for reference and illustrates the overall visibility of the eclipse (for an explanation of this map's features, see Key to Solar Eclipse Maps).

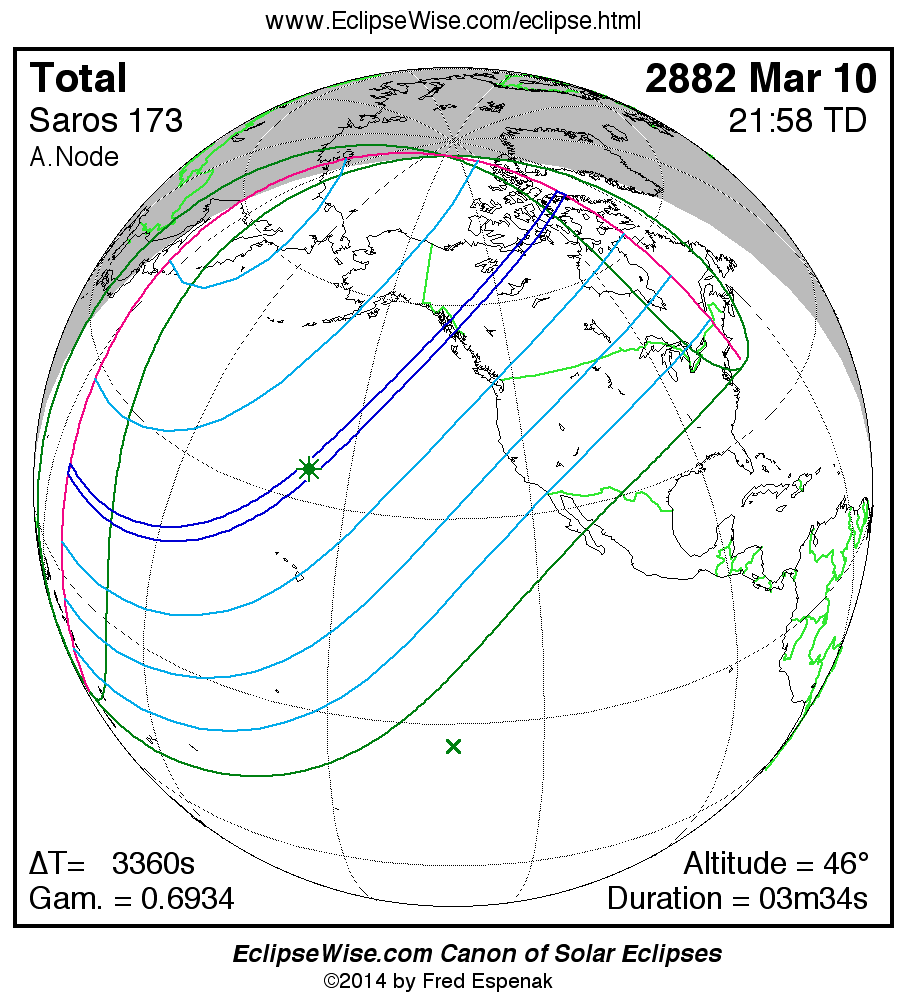
The interactive Google map below uses the Web Mercator projection to display the eclipse track. The large saddle-shapped region is the path of the Moon's penumbral shadow. This is the geographic region from which a partial eclipse can be seen. The northern and/or southern penumbral shadow path limits are plotted as green curves. The magenta loops represent the locus of all locations where the partial eclipse begins or ends at sunrise or sunset. Inside these regions the partial eclipse is in progress at sunrise or sunset. An orange curve also appears inside this region and represents the locus of all locations where the partial eclipse is at its maximum phase at sunrise or sunset. The Key to Google Eclipse Maps illustrates a map with each of these curves. You MUST be somewhere within the region enclosed by these curves in order to see the partial eclipse. Outside this region no eclipse is visible.

The narrow track running through rhe penumbral shadow is the path of the Moon's umbral shadow. This is the geographic region from which the total eclipse can be seen. The northern and southern path limits are blue and the central line is red. You MUST be somewhere within the central path (shaded zone framed by the blue lines) to see the total phase of the eclipse. The eclipse is longest on the central line (red). The yellow lines crossing the path indicate the position of maximum eclipse at 10-minute intervals.

The green marker labeled GE is the point of Greatest Eclipse. The magenta marker labeled GD is the point of Greatest Duration. This is the location where the total eclipse lasts the longest along the entire path. In this case, the Greatest Duration is 03m34.3s . This prediction does not take into account the mountains and valleys along the edge of the Moon. For the sake of speed and simplicity, the effects of the lunar limb profile are NOT used in the predictions and map presented on this page.

You can be hundreds of miles from the theoretical point of Greatest Duration and still enjoy totality lasting within a second of the maximum possible (as long as you stay within several miles of the central line).

Google Maps uses a close variant of the Mercator projection, and therefore cannot accurately show areas around the poles. As a consequence, eclipse paths at high latitudes will appear highly distorted and may degrade into a series of straight line segments.

Note: Your web browser must have Javascript turned on in order to see a solar eclipse path plotted on Google Maps. See compatible browsers for more information.
Map centered on (latitude, longitude):
Cursor position (latitude, longitude):
Distance from last marker: 		Show marker on click
 Large map

User Directions

The zoom buttons (the 'plus' and 'minus' buttons to the lower right corner of map) are used to change the magnification [3]. The user's cursor can be used for navigating around the map. Just hold the left mouse button down while the cursor is on the map, and drag the map around to reposition it. The two map buttons (top left) let you switch between map view and satellite view.

Click anywhere on the map to add a red marker. A popup window appears above the marker with the calculated eclipse times and duration of totality for that location (see explanation of Eclipse Circumstances below). The 'x' in the upper right corner of the popup window closes the popup window. Additional markers can be placed anywhere on the map. Move the cursor over a marker to reveal a popup window with the eclipse times for that position. The predictions in the popup window can also be displayed in a new web page via the Eclipse Times Popup button (just below the map and to the right). You can select and copy this infomation to paste into a word processor.

All the markers can be removed by using the Clear Marker button (below the eclipse map). Choose the Large Map check box to produce a bigger map (hint: enlarge the browser window to its maximum size before selecting the Large Map check box). This option is especially useful to users with large monitors.

Below the lower left corner of the map are three readouts. The first gives the geographic coordinates (latitude & longitude) of the map center while the second gives the geographic coordinates of the cursor. The third line gives the distance of the cursor from the last marker. For more information, see Google Eclipse Map Instructions.

Eclipse Circumstances

When you click on the map a red marker is added and a popup window opens giving the Eclipse Circumstances calculated for that location. The predictions in the popup window can be divided into two sections.

In the top part of the window, the decimal Latitude and Longitude of the marker are given. The Eclipse Type (either total, annular or partial) seen from that position is given. The duration of Totality (or duration of Annularity) lists the length of the total (or annular) phase in minutes and seconds. The Eclipse Magnitude is the fraction of the Sun's diameter eclipsed. The Eclipse Obscuration is the fraction of the Sun's area eclipsed.

The bottom part of the window consists of a table listing the times for important stages of the eclipse. The Event column lists eclipse phase, followed by the date and time (both in Universal Time). Finally, the Altitude and Azimuth of the Sun is given for each event. The altitude is measured from the horizon (0°) to the zenith (90°). The azimuth is measured from due North and rotating eastward (North = 0°, East = 90°, South = 180°, and West = 270°).

Important Note: The eclipse predictions in this interactive map DO NOT include the effects of mountains and valleys along the edge of the Moon. Such corrections for the lunar limb profile may change the contact times and eclipse durations by ~1-3 seconds. The exact location of Greatest Duration may also change by ~10-20 kilometers.

Footnotes

1. Other interactive Google eclipse maps include those by Xavier Jubier and Bill Kramer. ↩

2. An excellent source for weather prospects for upcoming eclipses is meteorologist Jay Anderson's Eclipsophile Eclipse Weather Page. ↩

3. This web page approximates the curved eclipse path by using a series or To maintain the validity of this approximation, the maximum zoom level is limited to ~1 mile/inch (~0.7 kilometers/centimeter). This should prevent over-interpretation of the eclipse path accuracy. You can disable the zoom limit using the link Maximum Zoom to reload the map. ↩

Links for the Total Solar Eclipse of 2882 Mar 10

Links to Additional Solar Eclipse Predictions

Calendar

The Gregorian calendar (also called the Western calendar) is internationally the most widely used civil calendar. It is named for Pope Gregory XIII, who introduced it in 1582. On this website, the Gregorian calendar is used for all calendar dates from 1582 Oct 15 onwards. Before that date, the Julian calendar is used. For more information on this topic, see Calendar Dates.

The Julian calendar does not include the year 0. Thus the year 1 BCE is followed by the year 1 CE (See: BCE/CE Dating Conventions). This is awkward for arithmetic calculations. Years in this catalog are numbered astronomically and include the year 0. Historians should note there is a difference of one year between astronomical dates and BCE dates. Thus, the astronomical year 0 corresponds to 1 BCE, and astronomical year -1 corresponds to 2 BCE, etc..

Eclipse Predictions

Predictions for the Total Solar Eclipse of 2882 Mar 10 were generated using the JPL DE406 solar and lunar ephemerides. The lunar coordinates were calculated with respect to the Moon's Center of Mass. Although the predictions are first calculated in Terrestrial Dynamical Time (TD), they are then converted to Universal Time (UT1) as presented here. The parameter ΔT is used to convert between the two times (i.e., UT1 = TD - ΔT). For this eclipse ΔT has a value of 3359.7 seconds. The uncertainty in ΔT is 752.5 seconds corresponding to a standard error in longitude of the eclipse path of ± 3.14°.

The eclipse predictions presented here DO NOT include the effects of mountains and valleys along the edge of the Moon. Such corrections for the lunar limb profile may shift the limits of the eclipse path north or south by ~1-3 kilometers, and change the eclipse duration by ~1-3 seconds.

Acknowledgments

All eclipse calculations are by Fred Espenak, and he assumes full responsibility for their accuracy.

Permission is granted to reproduce the eclipse data when accompanied by a link to this page and an acknowledgment:

"Eclipse Predictions by Fred Espenak, EclipseWise.com"

The use of diagrams and maps is permitted provided that they are unaltered (except for re-sizing) and the embedded credit line is not removed or covered.

Please visit the Acknowledgments Page for additional information.